[mechanics] Truss analysis: Practice

 

For a truss given below, can we solve the force acting on every member? Surprisingly, yes. Here’s how.

 

 

First, we have to solve the reactions on $H$ and $L$.

 

\begin{align*}
\Sigma M_{L} = 3Pa-4Ha = 0 \to& H= {3 \over 4} P
\\
\Sigma F_{y} = H+L-P=0 \to& L={1\over4}P
\end{align*}

 

 

Second, let’s get rid of zero-force members. For joint $D$ to be in equilibrium, $F_{DI}$ should be zero because there’s nothing to cancel it out on the opposite side. In a similar manner, $EI$, $FK$, $GK$ are also zero-force members.

 

 

Oh, it looks like we can do more. Members $AI$, $BJ$, $CK$ are free to go.

 

 

Removed members are force-free, but they still need to be in the truss to make the whole structure rigid. Remaining members are only the ones under tension or compression, but without the zero-force members they can not make a rigid truss. Interesting, right?

 

Third, I will use the method of joints because we want to get all the forces on members. Equilibrium of joint $H$ gives

 

 

\begin{align*}
\Sigma F_{y} = {F_{DH}\over\sqrt2} + {3\over4}P = 0 \to& F_{DH}=F_{AD} = {-3\sqrt2\over4}P
\\
\Sigma F_{x} = F_{HI} + {F_{DH}\over\sqrt2} = 0 \to& F_{HI} = F_{IJ}= {3\over4}P.
\end{align*}

 

Equilibrium on joint $A$ gives

 

 

\begin{alignat}{2}
\Sigma F_{y} &= {3\sqrt2\over4}P\cdot {1\over\sqrt2} - P - {F_{AE}\over\sqrt2} = 0 &&\to F_{AE}=F_{EJ}=-{\sqrt2\over4}P
\\
\Sigma F_{x} &= {3\sqrt2\over4}P\cdot {1\over\sqrt2} + F_{AB} + {F_{AE}\over\sqrt2}=0 &&\to F_{AB}=F_{BC}=-{1\over2}P.
\end{alignat}

 

Equilibrium on joint $J$ gives

 

 

\begin{alignat}{2}
\Sigma F_{y} &= -{\sqrt2\over4}P\cdot {1\over\sqrt2} + {F_{FJ}\over\sqrt2} = 0 &&\to F_{FJ}=F_{CF}={\sqrt2\over4}P
\\
\Sigma F_{x} &= -{3\over4}P + {\sqrt2\over4}P\cdot {1\over\sqrt2} + {F_{FJ}\over\sqrt2} + F_{JK}=0 &&\to F_{JK}=F_{KL}={1\over4}P.
\end{alignat}

 

Equilibrium on joint $L$ gives

 

 

\begin{alignat}{2}
\Sigma F_{y} &=  {F_{GL}\over\sqrt2} + {1\over4}P = 0 &&\to F_{GL}=F_{CG}=-{\sqrt2\over4}P
\end{alignat}

 

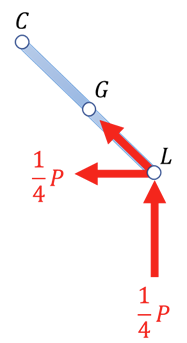
which confirms that

 

\begin{alignat}{2}
\Sigma F_{x} &= -{1\over4}P - {F_{GL}\over\sqrt2} = 0.
\end{alignat}

 

We didn’t check the joint $C$, but let’s make it sure.

 

 

\begin{alignat}{2}
\Sigma F_{x} &= {1\over2}P - {\sqrt2\over4}P\cdot {1\over\sqrt2}- {\sqrt2\over4}P\cdot {1\over\sqrt2}= 0
\\
\Sigma F_{y} &= - {\sqrt2\over4}P\cdot {1\over\sqrt2} + {\sqrt2\over4}P\cdot {1\over\sqrt2}= 0
\end{alignat}

 

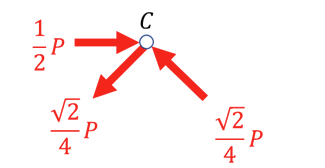
Phew, we made it.

 

- lazy engineer